Alice Kober vs Linear B, The World vs Voynichese…

by ⋅ 30 Comments

I’ve just finished reading @MargalitFox’s excellent book “The Riddle of the Labyrinth“, which untangles the skein of history around the decryption of Linear B to reveal the quiet (but huge) contribution made by Alice Kober.

Fox’s belief (which I largely agree with) is that Kober would, had she not died early, almost certainly have completed her decryption programme before Michael Ventris. Regardless, Ventris had spent years making a fool of himself by insisting loudly and at great length that the language of Linear B must surely be Etruscan (it was actually an early form of Greek, Δ’Ω!), and he only began making swift progress once he took Kober’s results on board.

Because Linear B was an unknown language written in an unknown script, Kober always insisted that anyone who took a theory about the language as their starting point was doomed to failure. Rather, the single route to the finishing line was, she asserted, to find the patterns and deep symmetries inside the primary texts that we have, and to work outwards from there.

Kober’s attempts to systematically comb through the Linear B texts were frustrated through the 1930s and part of the 1940s by Sir Arthur Evans’ refusal to release more than a modest fraction of them. However, she built up card indexes and added physical cross-referencing means (using carefully punched holes, she was able to optically find matching patterns, like using postcards to build her own Google search facility for Linear B ).

It is easy to draw a long list of comparisons between her sustained attack on Linear B and The World’s somewhat scattershot attacks on Voynichese. Perhaps unsurprisingly, few of these cast the latter in anything like a favourable light.

Similarities

Both Sir Arthur Evans and the Voyniches had very fixed (and, in retrospect, quite wrong-headed) ideas about the historical sources of their respective scripts / languages: and both released only a small number of images to scholars before their respective deaths.

Hence the constraints Alice Kober was working within during the 1930s and 1940s weren’t really so different from those that Voynich researchers ‘enjoyed’ for most of the 20th century. Her specific response was to make her own transcriptions, build her own analytical machinery, and construct her own decryption methodology.

If you want a direct apples-to-apples comparison, I’d perhaps suggest looking for the methodological parallels between Kober and Captain Prescott Hunt Currier (1912-1995). They both consciously and deliberately attacked their targets without a specific plaintext language in mind; discovered deep language-like patterns that nobody had either noticed or grasped the significance of; and then disseminated them openly.

Differences

The #1 difference is that while Linear B had Michael Ventris, Voynichese has had no Gary Lineker or Filippo Inzaghi hanging around on the goal-line to head Captain Currier’s critical cross into the goal.

While it’s easy to say that Ventris was brilliant, in many ways his whole approach to Linear B had been naive and self-defeating from the start. Margalit Fox concludes that Kober thought Ventris was yet another hacky Linear B amateur, far more of a research liability than a research asset: that he was so blinded by his idiotic Etruscan theory that his research would never (in fact, could never) produce anything of genuine value.

But Ventris’ key personal asset turned out to be that he had, as the famous US entrepreneur/investor Fred Wilson put it back in 2016, strong views weakly held. That is, once Ventris finally twigged that Kober had found something genuinely telling that was incompatible with his (previously strongly held) Etruscan theory, he had the strength of character to be able to jump ship completely. (Though admittedly Ventris did strongly hedge his initial description of what he had come up with by describing it as something that might be no more than a wonderful delusion.)

For me, the oddest thing about Voynichese is that even though modern researchers now know a vast amount about its inner workings (for example, you could hardly fault Torsten Timm’s diligence and persistence), they remain steadfastly unable to figure out the next step forwards.

If you can imagine a Voynichese football hanging in the air in front of goal while all the strikers are squabbling at the opposite end of the playing field, you’re not far off the truth. 🙁

Synthesis

Even though many now know about Linear B, what is less known is the story of Linear A. Also discovered by Sir Arthur Evans, the Linear A script is almost certainly a syllabary that was used on Crete to write a (now-lost) Minoan language. When the early Greek invaders came from the Mycenean mainland, they adapted Linear A as a script to write down (admittedly somewhat imperfectly) their Early Greek language.

Alice Kober realized early on that despite the many visual similarities between their sign shapes, Linear A and Linear B were writing down entirely different languages. Hence she abandoned all attempts to decrypt Linear A (because there were so few examples of it) and focused instead on the much more promising Linear B.

In many ways, we have a closely analogous situation with Voynichese, in that it comprises the two major ‘languages’ that Captain Currier identified in the 1970s. More recent research has identified even more subtlety to Currier’s A vs B division: the researcher Glen Claston (Tim Rayhel) asserted that he had identified the specific sequence by which Currier A evolved (or was actively mutated) into Currier B.

Even now, however, it remains absolutely the norm for researchers – even otherwise very good researchers – to carry out their analyses on the whole of their Voynichese transcription, i.e. all the A pages and B pages merged together into a single whole, as if they were all the same kind of thing.

Perhaps it should be no surprise that, to me, this is akin to mixing Linear A and Linear B into a single Linear corpus, superficial amateurish nonsense that Kober had nothing but disdain for in the 1930s and 1940s.

Hence if you genuinely want to be the Michael Ventris of Voynichese, I would suggest that you start by trying to learn from Alice Kober and Captain Currier:

  • Assume you know nothing at all about the unknown language(s) beneath the unknown script (because you don’t, you simply don’t)
  • Tackle one corpus at a time (say, Herbal A, Quire 13, or Quire 20)
  • Build up what you consider to be a reliable transcription for it
  • Build up contact tables
  • Begin with the patterns at the start, middle, and end of words
  • Determine the precise internal logic of the script, with the idea of working out how that might be interfering with the unknown language beneath that script

The missing Lonsdale Street nitkeeper…

by ⋅ 37 Comments

Let’s start with the original 26th Jan 1949 news story in the Adelaide News, the Sydney Daily Telegraph, the Geraldton Guardian, and the Age:

Melbourne.- Two prominent Melbourne baccarat players who desire to remain anonymous, believe they knew the unknown man in the “Somerton beach body mystery.”

They saw the man’s picture in a Melbourne newspaper and said they thought they recognised him as a “nitkeeper” who worked at a Lonsdale street baccarat school about four years ago. They could not recall his name.

They said the man talked to few people. He was employed at the baccarat school for about 10 weeks, then left without saying why or where he was going.

From a purely Melbourne-centric angle, the appeal for witnesses had only just appeared in the Herald (25 Jan 1949) and the Argus (25 Jan 1949). So it should be clear that the two baccarat players came forward immediately.

A few days ago, I wondered whether the man they had been thinking of might have been George Henry Newman. It’s true that Newman died in 1986, so could not have been the Somerton Man. However, given that people working at baccarat schools were generally younger than the Somerton Man, might the two baccarat players have mistaken George Henry Newman for the Somerton Man?

On balance, I think this is unlikely. Newman’s specific role in the whole baccarat ‘ecology’ was as a motor driver: that is, he would drive customers to and from the baccarat school. And he did that for years, not just for ten weeks.

So the person we’re looking for is someone quite specific and yet quite unusual (because of his age): a 40-year-old Lonsdale Street baccarat school nitkeeper circa 1945.

The annoying thing is that the Victorian police knew everything there was to know about these baccarat schools, but were unable to shut them down because of two specific factors:

  • The police had to catch punters while they were actually playing baccarat (and not other legal card games), which was actually quite a lot trickier than it sounds; and
  • The baccarat school principals bribed policemen left, right and centre to avoid the schools being shut down.

In the end, Christos Paizes’ long-running baccarat school got closed down courtesy of some kind of mealy-mouthed legal technicality, largely rustled up by Victorian politicians. And Paizes couldn’t really blame the poh-lice for that: they were too busy taking their brown envelopes to actual get him to court. Why would they ever call a halt on such a good thing?

The Missing Evidence

It seems that our well of Lonsdale Street baccarat school-related articles in Trove has now pretty much run dry. So where could we look next?

There is a ton of interesting stuff in the Victorian police archives – the NAA knows what it is, and wants to curate it, but nobody knows where it is. One day, this will come into the light, and perhaps there will be a sudden feeding frenzy from everybody with an interest in historical Melbourne crime. But… that blessed day still seems a way off just yet.

I wish I had found a list of Australian gamblers’ memoirs: anyone around Melbourne in the mid-1940s would have gone to Lonsdale Street, its baccarat Mecca. The closest I got was a series of brief articles in the Melbourne Argus of 1954, describing the memories of Melbourne baccarat school owner Robert Walker. (Incidentally, there’s a nice chapter on knockabouts in the 1986 book “Disorganized Crime”, which might still be available online.) Maybe there are more Aussie gamblers’ memoirs out there, please shout if you find any.

I therefore wonder whether the best thing to do would be to put an ad in a Melbourne newspaper – perhaps the Age, what do you think? – asking any lovely old people for their memories of Melbourne’s baccarat schools in the 1940s. Sounds like a Banker Bet to me… something to consider, anyway. 🙂

Any other suggestions for routes forward?

The difficulties of Australian archives…

by ⋅ 37 Comments

Towards the end of last year, I went through a period where I tried to finesse different ways of raking through the Australian archives to pursue the Melbourne nitkeeper research thread in the Somerton Man cold case. (Which arose because two Melbourne baccarat players came forward in early 1949 to say that they thought the man had worked as a nitkeeper at a Lonsdale Street baccarat school for about ten weeks around 1945 or so.)

Despite meticulously stepping through story after story in Trove to reconstruct what I could of the Melbourne baccarat school timeline, all I could come up with was the Romanian name “Balutz” that appeared in a single article. And when I received a nice reply from the Public Records Office Victoria helpfully suggesting I look up the (admittedly not entirely dissimilar) Greek surname Balutis, I then followed that lead as far as I could, all the way to Triantafillos Balutis and Stelios Balutis.

The pair (presumably brothers or cousins?) had arrived in Melbourne on 16 Feb 1923, both travelling in 3rd class on the RMS Ormonde: but I could find no details of what ultimately became of Triantafillos Balutis. He had lived for eight years in America (always a good box to tick for Somerton Man candidates, and the juicier the Juicy Fruit the better); had lived largely invisibly since 1930; had worked within a horse’s sneeze of the main Lonsdale Street baccarat school (which, let’s not forget, was run by the Greek Christos Paizes); and yet by around 1948 had completely disappeared from sight. No wife, no family, no funeral, no nothing.

In short, Triantafillos Balutis seemed pretty much a perfect candidate for having been the Somerton Man, if (and I know it’s very much an ‘if’) the Somerton Man had been the Melbourne nitkeeper the two gamblers claimed he was. But I was short of the last pieces of evidence that would confirm or deny it. What I needed was a cunning Aussie insider, a well-disposed researcher who would go to the Melbourne archives and ferret out the last pieces of the puzzle.

And that is indeed what happened next…

A Surreal Day Out At Shiel Street

The modern building at 99 Shiel Street, North Melbourne is shared by both the National Archives of Australia and the Victorian State Archives. According to my generous (but doggedly anonymous) Melbourne mole (I’ve lightly edited their notes):

The modern bureaucracy makes visiting here quite surreal. I’ll explain why in case you have reason to go to Shiel Street in future.

The first thing to note is that there is a single reading room, and along one side of it there runs a single long L-shaped desk.  

On the shorter side of the ‘L’ is a very nice librarian who hands over the National Archives Files.  As far as possible from her, at the very top of the long side of that ‘L’ is the very nice librarian who hands over files from the Victorian State Archives.

Now, if it happens that you speak first to the NAA librarian, he or she will tell you that there is no public digital scanning facility: in fact, only the librarians are allowed to do that, and they will charge you per scan.  Otherwise you can make ordinary photocopies at about three times you’d pay elsewhere… or you can bring a camera, or use your camera-phone.

However, if you happen to speak, instead, to the very nice Victorian Archives librarian at the other end of that long desk, you will be told that there’s no charge for anything. Not only that, but they have a dedicated side room (complete with professional-looking camera) where you are free to make hi-res digital scans if you prefer.  All for no charge whatsoever. “All you need to bring is a USB stick” they will tell you over the phone.

And, oddly, neither of the nice librarians is wrong.

It seems that the commonwealth (=Federal) government won’t agree to let its records be snapped using Victorian government equipment, so if you turn up with just a USB stick for NAA docs, you’ll be out of luck.

Luckily I had both a usb and a phone… which is why I’m sending through a neat pdf of the (Victorian Archive) probate material and a whole lot of poorly-lit camera snaps of the really interesting NAA material.

Needless to say, I’m extremely grateful for the kindness this anonymous researcher hero showed.

Stelios Balutis

We can now say a little more about what happened to Stelios Balutis. In his July 1963 will, the (obviously misspelled) “Stelois Balutes” of 581 King Street West Melbourne did:

“[…] Give devise and bequeath all my Estate both real and personal unto my Trustees Upon Trust […] for my granddaughter ELEONORA ASSIKIS of Sinikismos Evangelistries Ano Skalakia Thessaloniki Greece if she attains the age of Twentyone years and if living at my death but she shall predecease me then Upon Trust for my grandson NIKOLAS ASSIKIS of the same address if he attains the age of Twentyone years and if living at my death.”

His estate amounted to $1381.15: and the notice of probate appeared in the 02 Sep 1977 edition of the Melbourne Age. The only thing I rather liked was the colour of the probate’s duty stamps (in the NAA scan):

The Victorian Archives had more about Stelios Balutis. I’ll spare you his fingerprints, but there was a perfectly nice photo of him from his 1948 passport (which I contrast-enhanced slightly for clarity):

All in all, nothing remarkable, then; but even so, more than enough to close our (admittedly small) chapter on Stelios Balutis.

Triantafillos Balutis

Because I had previously been able to access Triantafillos Balutis’ application for Australian naturalization via the NAA’s website (my attempts to do this were mainly hindered by the 20+ different spelling combinations of his first and last names), our Melbourne mole was able to find only a single page of additional information in the archives.

Luckily (or possibly unluckily, depending on your Somerton Man point of view), this was the most important page of all, because it revealed what ultimately became of him. This was from the Criminal Investigation Board, whose (small) file relating to Triantafillos Balutis’s naturalization was included separately in the NAA records.

At the end, the file noted: “Triantafillos BALUTIS appears on Passport List No. 2857 of 15/9/1949 Proceeding to Greece. CIB.”

Ships leaving Melbourne for Europe on the 15th September 1949 were (according to Trove) the Devon for London and the Port Vindex for Liverpool, or (on the 16th) the Dundalk Bay for Adelaide and Naples.

By far the most interesting one of these was the Dundalk Bay, which had just arrived from Naples accompanied by the Nelly, the two ships carrying more than a thousand migrants each from all over Europe.

The Australian archives contain nominal rolls (all nicely digitized and cross-referenced) listing all the incoming migrants for the Dundalk Bay and Nelly (in fact, these lists appear several times over). But as far as I can see, there is no sign of nominal rolls for passengers travelling in the opposite direction: presumably because nobody in their right mind would want to be going back to Europe in September 1949.

As a result, I wasn’t able to dig up anything as useful as a nominal roll for any of the three ships listed as leaving on the 15th/16th September 1949, to fully confirm the (already extremely likely) story that Triantafillos Balutis left Melbourne for Europe then.

Perhaps someone with better m4d archival sk1llz than me will be able to dig this up. But to be fair, there’s probably little point: this research strand seems to have also reached the end of its life. We’re done here, basically.

So… Back To Lonsdale Street, Then?

I’ve been thinking about this whole thing for a couple of months now, in a kind of methodological post mortem. And I think the way it all rolled out revealed weaknesses in the way I was approaching archival research. In essence, I jumped at the chance to pursue what (superficially) seemed like a substantial lead, because it seemed likely that I would be able to follow a research lead on a single person of interest right to the end line (which is indeed what happened).

Sure, this was a plausible (if slightly opportunistic / optimistic) plan, but at the same time it didn’t really amount to anything like a systematic, goal-directed attack on the archives. And in fact this was what was missing.

So, in retrospect what I should have done was try to devise ways to open up the Australian archives in respect of the Lonsdale Street baccarat schools, and particularly the Victorian police records. We know (thanks to the PROV) that there was nothing Balutz-related in the Victorian Police Gazette for 1944/1945/1946: but Balutz should only ever have been a helpful secondary angle to prise open the archival lid.

Because Christos Paizes was the big fish in the story, I now think it was Paizes’ Melbourne history that needed bringing into the light in a far more systematic way, rather than guessing and hoping.

Christos Paizes and his Henchmen…

The NAA records say that Christos Paizes was born on 5th February 1897 in Ithaca, Kionion, Greece: and that he arrived in Australia on 4th January 1914. His naturalization was in 13th August 1937, at which time his address was (the familiar-sounding address) 269-271 Lonsdale-street, Melbourne.

According to the sensational (but probably not entirely historically reliable) book “Gangland Melbourne“, Paizes (also known as ‘Harry Carillo’) allegedly had Freddie ‘The Frog’ Harrison and Norman Bradshaw ‘working’ for him. All the same, Harrison was mentioned quite openly here as having worked for Solomon’s baccarat school, so I’m not yet convinced that Gangland Melbourne completely nailed that one:

Police witnesses alleged Harrison was the constant companion of thieves, that as doorkeeper of a baccarat school in Elizabeth-street, city, he had many times given warning of the approach of police, and, that he had kept a supply of bullets in his home. Harrison said he was employed by the proprietor, Mr. Solomon, as doorman of the Rendezvous Bridge Club, until May 20. From a weekly wage of £5 he paid £3 board to his aunt, with whom he and his wife and child had been living for twelve months. He had nothing to do with the conduct of the bridge club.

He was also mentioned quite openly in this news story on Trove:

Described by detectives as former doorkeeper for a baccarat school mentioned in connection with an Elizabeth Street shooting on May 22, Frederick William Harrison, 26, of Peel Street, Windsor, laborer, successfully appealed to Judge Mitchell in General Sessions today against a three months’ gaol sentence for vagrancy.

This was the baccarat school in Fink’s Building, according to this report.

Even though Harrison was not convicted of the Elizabeth Street shooting, George Henry Newman (45) was, and in October 1947 went to jail for two years. There’s a picture of Newman in an article in the Sydney Truth, which to my eyes isn’t terrifically different from the Somerton Man:

There’s no details of when Newman was let out of jail: and Trove has no obvious further trace of him. Just sayin’, just sayin’… 😉

We know that Christos Paizes subsequently surfaced in Sydney, running (no surprises) a baccarat school there. According to the Sydney Crime Museum, (quoting the 1980 book Drug Traffic by Alfred McCoy, which – with the inevitable shipping from Australia – is currently sitting just outside my comfortable price range, though the British Library does have a copy) when casinos in the 1970s became the next ‘hot’ place for gamblers to go to:

The established Goulburn Club at 51-7 Goulburn Street, owned by George Zizinos Walker and Christos Paizes of South Coogee, simply added roulette to baccarat, recruited a bevy of hostesses, and polished up its image. 

Putting all this together: it seems to me that if the Somerton Man was in some way connected with the Lonsdale Street baccarat schools, a far better first research step would be to map out the different Melbourne schools and all the crims and thugs associated with them, and only then with that groundwork in place start to look at individuals.

Back to the Archives?

And so the actual research question finally arrives: what is the best way of using Australian archive resources to try to reconstruct the Lonsdale Street baccarat school crim network circa 1945? After all, historians now spend so much of their time mapping out social networks, why not map antisocial networks too? :-p

Hence I think it’s the NAA’s B745 series that perhaps offers us the possibility of some kind of way in. However, when our marvellous Melbourne mole specifically asked the NAA about getting access to B745, the response was:

With regards to series B745, ‘Index of offenders investigated by the Commonwealth Police’, this is a collection of index cards which the National Archives does not have in custody. Although the series is registered with us it does not appear to have ever been transferred from the Australian Federal Police. Theoretically it may still be held by them, but previous attempts to identify their whereabouts have not been successful.

And so, for a change, one research door shuts only for another to also shut. There must surely be a way of locating B745 but… it will probably take a while.

Nick’s Challenge Cipher #1 cracked by Louie Helm…

by ⋅ 2 Comments

From 1991 onwards, John Walsh – the well-known host of “America’s Most Wanted” – received a series of ominous and threatening letters signed “SCORPION”. Some of these contained a series of Zodiac-style homophonic ciphers: to date, only two of these have been released. Unsurprisingly, these are known as the “Scorpion Ciphers“: but none has yet been cracked.

However, there’s reason to believe (as I pointed out in 2017) that there are some specific regularities with the S1 (‘Scorpion #1’) ciphertext and even more so with the S5 ciphertext that we might be able to exploit. Even though both ciphers at first sight resemble the Zodiac Killer’s ciphers, the Scorpion’s seem to cycle between specific sets of homophonic symbols. In the case of the S5 ciphertext, the cycling seems particularly rigid, in that it cycles between 16 alphabets.

Cryptologically, the problem was that ‘traditional’ homophonic solvers (such as Jarlve’s excellent AZDecrypt) have no way to include extra cipher system constraints, regardless of how stringent they may be. For example, it would be a reasonable hypothesis that S5 uses only a single alphabet for each of its 16 columns: but this is not something that any current solver could use.

After a fair bit of thought, I came up with the idea of putting out a set of challenge ciphers using a completely rigid cycling homophonic cipher, to try to spark interest in solving this class of ciphertext. And so, also back in 2017, I posted up a page containing seven constrained homophonic challenge ciphers. Despite a shockingly high bounty of £10 being on offer for the best solve by the end of 2017, nobody managed to grab my cash.

http://ciphermysteries.com/wp-content/uploads/sites/6/2017/06/smiley-10-pound-note.jpg

Had I made even the longest challenge cipher far too difficult? Would nobody ever solve these? Despite my doubts, I remained reasonably confident some clever person would find a way in, sooner or later…

2020: Enter Louie Helm…

And so it was with great delight that I received an email this morning from Louie Helm, asking me to check his solution. As a recap, my challenge cipher #1 (neatly arranged into its five columns) was:

121,213,310,406,516,
108,200,323,416,513,
112,208,308,409,515,
102,216,309,425,509,
114,215,309,417,507,
102,201,323,401,517,
111,200,306,408,500,
113,203,313,407,512,
103,223,313,403,511,
119,213,316,416,511,
102,204,324,418,517,
120,203,324,407,516,
105,209,312,401,504,
117,208,310,408,500,
113,203,301,425,513,
115,201,313,408,515,
115,214,308,406,501,
122,204,322,408,509,
114,209,305,412,504,
117,213,316,402,509,
100,200,310,423,513,
100,214,320,419,509,
114,209,309,419,520,
101,200,320,416,518,
120,211,313,403,509,
103,207,313,421,513,
107,209,305,407,523,
115,224,313,416,508,
102,203,306,416,514,
107,200,310,401,509,
103,212,324,

Louie’s claimed plaintext was:

THEOBJECTOFMYPROP
OSEDWORKONCYPHERI
SNOTEXACTLYWHATYO
USUPPOSEBUTMYTIME
ISNOWSOENTIRELYOC
CUPIEDTHATIHAVEBE
ENOBLIGEDTOGIVEIT
UPATLEASTFORTHENE
XTTWOORTHREEYEARS

…which was completely correct! Fantastic work, well done!!! 🙂

(Extra crypto brownie points on offer for anyone who recognizes the – admittedly somewhat obscure – source of the quotation.)

Crypto-fans may well recognize Louie’s name as having been (along with Jarlve) one half of the recent pair of solvers of Klaus Schmeh’s 1000-bigram (and then even harder 750-bigram) challenge. And here’s Louie’s cool-looking photo:

So: one last time – well done, Louie, you rock. 😉

So… How Did Louie Do It?

This is, of course, the interesting question: and Louie answered it in a forum post on zodiackillersite.com on 02 Jan 2020, which I can do no better than simply quote in full:

I solved it with AZdecrypt v1.17 using my newest 8-gram model released a few days ago on Christmas. The only modification I made was adding 15 lines of code to restrict the solver to only use one homophone for each of the five columns. The solve for cipher #1 succeeded, but the way I modified the solver to do it is very inelegant since it can quickly lock high-scoring letters into place and then deprives the hill climber of further opportunities to test them in other arrangements. A more well-tuned version of this general solution would merely penalize (but not forbid) repeated letters in each column. This would allow the solver to evolve through a less jagged solution landscape and then still eventually arrive at a 1-homophone/column solution in the end. I predict this sort of modification would likely solve cipher #2 and beyond.

Although I used the column constraint (and it appears to have been necessary for a quick solve), the larger story here is probably the recent improvements to AZdecrypt and the release of 8-gram models in 2019.

For instance, if you simply ignore Nick’s constraint and use Jarl’s state of the art solver + his best n-gram file from 2018, you would have needed an 8 word crib to quickly decrypt challenge cipher #1:

AZdecrypt v1.14 + 7-gram jarl reddit: THEOBJECTOFMYPROPOSEDWORKONCYPHER

But in 2019, Jarl moved away from his IoC-based solver to a more capable entropy-based one. This alone drops the required crib needed to solve the cipher down to 2-3 words:

AZdecrypt v1.17 +
6-gram jarl reddit: THEOBJECT — PR — CYPHER
7-gram jarl reddit: THEOBJECT — PR

And doing the same execrise with the n-gram models I’ve released during 2019 shows they progressed from needing an 8 word crib — down to just a 5 letter crib:

AZdecrypt v1.17 +
6-gram v2 (May 2019): (no cribs sufficient)
6-gram v3 (Jun 2019): THEOBJECTOFMYPROPOSEDWORKONCYPHER — ~90% correct solve
6-gram v4 (Oct 2019): THEOBJECTOFMYPROPOSEDWORKONCYPHER — 100% correct
6-gram v5 (Dec 2019): THEOBJ — PR — CYPHER

7-gram v2 (May 2019): THEOBJECT — PR — WORK
7-gram v3 (Jun 2019): THEOBJECTOF — WORK
7-gram v4 (Oct 2019): THEOBJECT — WORK
7-gram v5 (Dec 2019): THEOBJ — WORK

8-gram v2 (May 2019): THEOBJECT — WORK — CYPHER
8-gram v3 (Jun 2019): THEOBJ — WORK — YPH
8-gram v4 (Oct 2019): THEOBJ
8-gram v5 (Dec 2019): HEOBJ

Note: These are just the cribs I got to work in under a minute using a completely unmodified version of AZdecrypt. It’s quite possible that any of us using either of the last two version of AZdecrypt + beijinghouse 8-gram files could have solved this since Oct 2019 simply by letting it run long enough.

So the real story here seems to be that to crack my first challenge cipher, the three things that were necessary were not only Louie Helm’s tweaks to AZDecrypt to exploit the column constraints, but also Jarlve’s huge improvements to AZDecrypt’s homophonic solver during 2019, along with Louie’s now very extensive 8-gram files.

I think this is a great result for Louie (and for Jarl too!), and I have nothing but admiration and applause for the pair of them. Rock and roll, guys!

The (Inevitable) Crypto Punchline…

Of course, challenge cipher #1 was (numerically) the easiest one, in that it was the longest of the set. So the big test will be to see how far through the list of challenge ciphers Louie Helm’s approach will (admittedly with a bit of tidying up) now be able to reach.

Interestingly, Louie immediately noted that my challenge cipher #2 presents some obvious-looking crypto weaknesses:

  • the 1st and 10th lines (of five symbols each) are identical
  • the 4th and 19th lines are also identical, and share three consecutive symbols with line #22.

He then speculates that it might be worth attacking these patterns in cipher #2 using common words or phrases such as “THERE” / “THOSE” / “I HAVE” / “IN THE” / “IS THE” / “IT WAS”.

Personally, I haven’t looked at the plaintexts since I enciphered them two and a half years ago (and I have no intention of doing so until such time as a proposed decryption arrives here), so I’m not going to be much help. 😉

The only information I’d add is that I took each of the seven short texts from completely different places: so knowing the source of “The object of my proposed work on cypher…” shouldn’t directly assist with the others.

But what I want to now say is: good luck, everyone! The game is afoot!

A Father Christmas attack on the Voynich Manuscript…?

by ⋅ 30 Comments

It’s that time of year when a Voynich researcher’s mind turns to life’s most important questions. Such as whether it is possible to use Father Christmas to decrypt the Voynich Manuscript.

For a start, it’s entirely possible that there is Christmas-related imagery hidden in plain sight in the Voynich Manuscript, but we’ve just been too distracted by the details to notice them:

Before I go any further, I should say that I do know full well that what we now think of as ‘Santa Claus’ was in fact a 19th century faux-historical mash-up of loads of other stuff, and that he originally wore green clothes (not red). But I would – as spin doctors now tell us all the time – say that, wouldn’t I?

All the same, it’s probably safe to say that we would have zero luck using a 19th century cultural crossover to decrypt a 15th century object. However, we might have more luck with the layer that preceded him – by which I mean St. Nicholas.

This might be interesting because the zodiac roundel drawings in the Voynich Manuscript’s ‘zodiac’ section bear a strong resemblance to the zodiac roundel drawings found in early fifteenth century Alsace calendars (specifically 1420-1430, it’s all in the sleeves and the necklines). Hence I think there’s a reasonably good chance that what we’re looking at there is some kind of calendar – and the most important details written on calendars were feast days celebrating local saints.

If this section is indeed some kind of calendar (and it’s still speculation, remember), there’s a decent chance it was arranged not by zodiac degree but by month. But what day did the year start on in the 15th century?

Back then, this was not universally 1st January, not at all. In fact, as Rafal Prinke pointed out in 2001 (I quoted him in 2009), the Venetian year instead started on 1st March, while the Florentine year started on 25th March. The reason this is relevant is that the first zodiac sign depicted on a Voynich zodiac roundel is Pisces, which (though it astrologically / zodiacally starts in late February) was typically associated with March. (We are sufficiently certain of the folio order that we can be sure Pisces came first.)

So let’s make today’s educated guess: that the Voynich ‘zodiac’ section is actually a calendar of feast days that starts on 1st March. (It might even be some kind of wonky Cisioianus, nobody knows.) Does that give us a Father Christmas attack on the Voynich Manuscript?

Well… it might do. On the page for (according to our guess) December (it has a Sagittarius crossbowman roundel drawing in the centre), there are thirty ‘labels’ (attached to the thirty ‘nymphs’). And one of these labels might just be saying St. Nicholas, right?

As an aside: to my eyes, there are plenty of annoying (or at least slightly unsettling) details on this page:

  • It seems that the labels were added in a different ink and by a different quill
  • It seems that most (but not all) of the nymphs’ breasts were added by that same different hand
  • Some of the nymph outlines were also updated with that same quill
  • There’s a particularly badly drawn barrel outline added behind the top-left nymph just outside the largest ring
  • There’s green paint contact transfer from the facing page BUT that would seem to imply that the now-missing folio immediately afterwards (Capricorn and Aquarius) was not there when the green paint was added. Which would seem to imply that the green paint (at the very least) was added some time (probably a century or more?) after the initial composition phase(s).

But, getting back to Cisioianus (feast day mnemonics), a German 15th century Latin version for December run as follows:

  • December Barba Nico Concep et alma Lucia
  • Sanctus abinde Thomas modo Nat Steph Jo Pu Thome Sil.

We can decode this syllable by syllable to reveal the list of feast days that the mnemonic was trying to help people memorize (with a little help from Grotefend’s 1891 “Zeitrechnung des deutschen Mittelalters“, p.35):

  1. De-
  2. cem-
  3. ber
  4. Bar- – Feast of St Barbara
  5. ba
  6. Ni- – Feast of St Nicholas
  7. co
  8. Con- Feast of the Immaculate Conception
  9. cep
  10. Et
  11. Al-
  12. ma
  13. Lu- – Feast of St Lucy
  14. Ci-
  15. a
  16. Sanc-
  17. tus
  18. Ab-
  19. in
  20. de
  21. Thom- – Feast of St Thome Ap
  22. as
  23. mo-
  24. do
  25. Nat – Nativ. Domini
  26. Steph – Feast of St Stephen
  27. Jo
  28. Pu
  29. Tho- – Feast of St Thome Asp (St Thomas a Becket)
  30. me
  31. Sil – Feast of St Sylvester

What immediate emerges is that if we’re looking for St. Nicholas in the 15th century, his feast day was actually on December 6th, neatly sandwiched between St Barbara (December 4th) and the Feast of the Immaculate Conception (December 8th).

Hence if the text on this page is some kind of Cisioianus mnemonic for December, we might hope to find labels in a sequence that looks vaguely like “Bar Ba Ni Co Con“. Now, I personally can’t see anything there that quite fits this pattern at all. But that doesn’t mean it isn’t there. 😉

But, sadly, this is just about as far as St Nicholas’ Christmas sleigh can carry us into the speculative world of Voynich research. Happy Christmas to you all! 🙂

Pavia MS Aldini 488: “Collectio de Balneis”…

by ⋅ 38 Comments

When a comment landed here today from Diane O’Donovan about the (sometimes asserted, sometimes denied) connection between the Voynich Manuscript’s Q13 (the ‘balneo’ folios) and the late 12th century De Balneis Putolanis by Peter of Eboli, it reminded me that there’s a 15th century balneological manuscript out there I really want to know a lot more about – MS Aldini 488 “Collectio de balneis”.

Q13A vs Q13B (again)

However, before I launch into all that, I first need to recap various codicological features of Q13 before we start trying to work with it.

The first thing to know about Q13 is that its bifolios have ended up bound in the wrong order. We can tell this because a bifolio that was originally at the centre of a quire / gathering has ended up not at the middle. Moreover, following the logical through to the end leads (as per The Curse of the Voynich back in 2006) to a situation where you can reconstruct the central two bifolios’ nesting order: f84 – f78 (centre) f81 – f75.

The second thing to notice is that the drawings on these two nested Q13 bifolios (which are all about bathing ‘nymphs’) seem to sit in a quite different category from the drawings on the other three Q13 bifolios (which largely revolve around plumbing, though whether this is real or imagined is hard to say). Voynich researcher Glen Claston proposed that the first two bifolios form a balneological quire on their own (which he called “Q13B”), while the other three form a medical quire (which he called “Q13A”). Regardless of whether you agree or disagree with his specific interpretation, his basic codicological division into two separate artifacts has stood the test of time: so it seems we are looking at two separate (though similar-looking) things whose constituent bifolios have ended up interleaved. Glen also proposed that Q13B was constructed before Q13A.

The third thing to be aware of is that on the Voynich Manuscript’s page f81r (in Q13B, the bathing nymphs section), there is apparently a poem. I raised this poem section as something which we might look for parallels with in other texts when I started discussing the ‘block paradigm’ approach (where you look for structural matches between a page of enciphered text and plaintext pages from similar contemporary or earlier manuscripts). Interestingly, it seems (from the line-initial gallows characters) that the f81r poem has a 7 / 8 / 8 / 8 line structure, which would be consistent with the writer / copyist having accidentally skipped past a line within the first block of the poem.

Putting all these pieces together, the implication is that we should be looking for a block-sized match between the contents of the two “bathing nymphs” bifolios and 14th / early 15th century balneological texts (which are possibly but not necessarily illustrated). The poem embedded in the middle (it’s on the right half of the central bifolio) seems to be structured as three verses, each containing four couplets (i.e. eight lines). This is because if we can find a source match that’s tolerably close to this basic ‘block specification’, we might just be in business.

Arnold C. Klebs

Back when I was writing The Curse of the Voynich in 2006, to be honest I hadn’t yet found much balneological source material at all. It was only a little later (in 2009) that I found an online version of the (1916) article “Balneology in the Middle Ages” by Arnold C. Klebs. This mentioned a number of late medieval / early modern people who had written on the subject of baths, e.g.:

  • Giovanni de Dondis
  • Gentile da Foligno (d. 1348)
  • Ugolino Caccino of Montecatini (d. 1425)
  • Matteo Bendinelli (1489)
  • Michele Savonarola (who I already knew about)

Klebs also mentioned the printed book “De Balneis omnia quae extant” Venice, Giunta, 1553, fol., 447 leaves, which he describes as “the first text-book on balneology“.

A source Klebs also refers to specifically for German bath history is:

  • Martin, Alfred “Deutsches Badewvesen in vergangenen Tagen,” Jena, Diederichs, 1906. With 159 illustrations from old originals.

Giunta’s (1553) De Balneis Omnia

Google Books lists two separate copies of Giunta’s (1553) “De Balneis Omnia Quae Extant apud Graecos, Latinos et Arabas” (etc etc), both of which freely downloadable:

The bad news is that this is a super-heavyweight Latin compendium of balneological sources (the PDF runs to 1033 pages). However, the good news is that Giunta has assembled it from just about everyone pre-1553 who had written about baths, water etc: so there are large sections excerpting books from early authors such as Pliny, Avicenna, Aristotle, Galen, Hippocrates, along with selections from 16th century authors such as Gesner, Fuchs etc.

One thing I found was that, Pietro d’Abano’s “De Balneis” aside (which is written in hexameters), almost none of the balneo sources quoted by Giunta seem to appear in verse form. Even the promising-looking verse section on p.90 by “Ioannis et Iacobi De Dondis Patavinorum” turned out to be a poem by Claudian (370AD-404AD) (“Fons, Antenoreae vitam qui porrigis urbi, / Fataque vicinis noxia pellis aquis“).

Having said that, Giunta’s selection is entirely in Latin and far from complete. So it may well be that, if we can somehow go back to some fifteenth century collection of balneo manuscripts, we can see these in their original form – which may well be in Tuscan (rather than Latin), in verse (rather than in prose), and illustrated (rather than just text).

But where on earth would we find such an unlikely-sounding text?

Pavia, MS Aldini 488

It turns out that there really is such a text: and it is at the University of Pavia. Sadly, this “Collectio de Balneis” hasn’t yet been digitized (or if it has, its digital pages have not yet been shared outside the University of Pavia). But we do know the contents of MS Aldini 488:

Aldini 488, Collectio de Balneis. Cart., sec. XV, cc. 78 n.n., 232 x 154 mm.
c. 1: Savonarola Michele, De balneo et termis naturalibus omnibus Italiae sicque totius orbis proprietatibusque earum
c. 45: Ugolino da Montecatini, De balneis mineralibus et artificialibus
c. 61: Epigrammata de balneis puteolanis
c. 66: Consilium pro balneis de Corsena in comitatu luchano pro domino Lanzaloto de Crotis ducali consiliario
c. 67: Tura di Castello, Regula et tractatus balnei de poreta
c. 68v: Tractatus pro balneis de aquis per Petrum de Tussignano
c. 70v: Antonii Guaynerii papiensis de balneis aquis ciuitatis antiquissime que in marchionatu montisferrati sita sunt tractatus
c. 74v: De balneis secundum Petrum de Ebano
c. 75v: Tractatus de balneis secundum Gentillem
c. 76v: De balneis de Burmio secundum magistrum Petrum de Tussignano
c. 77v: Regula balnei loci de Aquaria in territorio regii
c. 78v: De balneo aque porrete
Collocazione cd: Mediateca nas bu 269
Collocazione vol. originale: Aldini 488

Because this is not yet available online, it is where – unless you happen to know better? – our current breakneck tour of balneological sources stops,

Note that there are a fair few monographs on individual balneo authors:

  • This Spanish Prezi presentation by Sergio P on Michele Savonarola lists eight manuscript versions of his book on baths (including Paris BNF Nouv. Acq. Lat 889, dating to 1452), seven printed versions of the same (1485-1562), plus four separate monographs.
  • Pietro da Tossignano’s (d. 1401) “Tractatus de regimine sanitatis” was printed in 1535 (his medical recipes are online here). Giuseppe Mazzini wrote “Vita e opera di maestro Pietro da Tossignano” in 1926 (reprinted in 2007).

As an aside, I also found an interesting chapter (in French) on the balneo literature – “Les traités médicaux sur les bains d’Acqui Terme, entre XIVe et XVIe siècles“, by Gabriella Zuccolin – from a recent book, “Sejourner au bain”. Zuccolin further notes that many of the treatises are covered at speed by Lynn Thorndike, but… they would be, wouldn’t they?

Voynich Manuscript: Letter Contact Tables

by ⋅ 28 Comments

A recent post on voynich.ninja brought up the subject of differences / similarities between Voynichese words starting with EVA ch and those starting with EVA sh. But this got me thinking more generally about the difference between ch and sh in Voynichese (i.e. in any position), and even more generally about letter contact tables.

Problems With Letter Contact Tables

For ciphertexts where the frequency instance distribution has been flattened, a normal first test is William Friedman’s Index of Coincidence (IoC). This often helps determine the period of the cryptographic means that was used to flatten it (e.g. the length of the cyclic keyword, etc). But this is not the case with the Voynich Manuscript.

For ciphertexts where the frequency instance graph is normal but the letter to letter adjacency has been disrupted, the IoC is one of the tests that can help determine the period of any structured transposition (e.g. picket fence etc) that has been carried out. But the Voynich is also not like this.

So, when cryptologists are faced by a structured ciphertext (i.e. one where the frequency instance graph more closely resembles a natural language, and where the letter adjacency also seems to follow language-like rules, the primary tool they rely on is letter contact tables. These are tables of counts (or percentages) that show how often given letters are followed by other given letters.

But for Voynichese there’s a catch: because in order to build up letter contact tables, you have to first know what the letters of the underlying text are. And whatever they might be, the one thing that they definitely are not is the letters of the EVA transcription.

Problems With EVA

The good thing about EVA was that it was designed to help Voynich researchers collaborate on the problems of Voynichese. This was because it offered a way for them to talk about Voynichese that online was (to a large degree) independent of all their competing theories about what specific combinations of Voynichese shapes or strokes genuinely made up a Voynichese letter. And there were a lot of these theories back then, a lot.

To achieve this, EVA was constructed as a clever hybrid stroke transcription alphabet, one designed to capture in a practical ‘atomic’ (i.e. stroke-oriented) way many of the more troublesome composite letter shapes you find in Voynichese. Examples of these are the four “strikethrough gallows” (EVA ckh / cth / cfh / cph), written as an ornate, tall character (a “gallows character”) but with an odd curly-legged bench character struck through it.

However, the big problem with EVA is arguably that it was too successful. Once researchers had EVA transcriptions to work with, almost all (with a few heroic exceptions) seem to have largely stopped wondering about how the letters fit together, i.e. how to parse Voynichese into tokens.

In fact, we have had a long series of Voynich theorists and analysts who look solely at Voynich ‘words’ written in EVA, because it can seem that you can work with EVA Voynichese words while ignoring the difficult business of having to parse Voynichese. So the presence of EVA transcriptions has allowed many people to write a lot of stuff bracketing out the difficult stuff that motivated the complicated transcription decisions that went into designing EVA in the first place.

As a result, few active Voynich researchers now know (or indeed seem to care much) about how Voynichese should be parsed. This is despite the fact that, thanks to the (I think somewhat less than positive) influence of the late Stephen Bax, the Voynich community now contains many linguists, for whom you might think the issue of parsing would be central.

But it turns out that parsing is typically close to the least of their concerns, in that (following Bax’s example) they typically see linguistic takes and cryptographic takes as mutually exclusive. Which is, of course, practically nonsensical: indeed, many of the best cryptologists were (and are) also linguists. Not least of these was Prescott Currier: I would in fact go so far as to say that everyone else’s analyses of Voynichese have amounted to little more than a series of minor extensions and clarifications to Currier’s deeply insightful 1970s contributions to the study of Voynichese.

Problems With Parsing

Even so, there is a further problem with parsing, one which I tried to foreground in my book “The Curse of the Voynich” (2006). This is because I think there is strong evidence that certain pairs of letters may have been used as verbose cipher pairs, i.e. pairs of glyphs used to encipher a single underlying token. These include EVA qo / ee / or / ar / ol / al / am / an / ain / aiin / aiiin / air / aiir (the jury is out on dy). However, if you follow this reasoning through, this also means that we should be highly suspicious of anywhere else the ‘o’ and ‘a’ glyphs appear, e.g. EVA ot / ok / op / of / eo etc.

If this is even partially correct, then any letter contact tables built on the component glyphs (i.e. the letter-like-shapes that such verbose pairs are made up of) would be analysing not the (real underlying) text but instead what is known as the covertext (i.e. the appearance of the text). As a result, covertext glyph contact tables would hence be almost entirely useless.

So I would say that there is a strong case to be made that almost all Voynichese parsing analyses to date have found themselves entangled by the covertext (i.e. they have been misdirected by steganographic tricks).

All the same, without a parsing scheme we have no letter contact tables: and without letter contact tables we can have no worthwhile cryptology of what is manifestly a structured text. Moreover, arguably the biggest absence in Mary D’Imperio’s “An Elegant Enigma” is the lack of letter contact tables, which I think sent out the wrong kind of message to readers.

Letter Contact Tables: v0.1

Despite this long list of provisos and problems, I still think it is a worthwhile exercise to try to construct letter contact tables for Voynichese: we just have to be extraordinarily wary when we do this, that’s all.

One further reason to be wary is that many of the contact tables are significantly different for Currier A and Currier B pages. So, because I contend that it makes no sense at all to try to build up letter contact pages that merge A and B pages together, I present A and B separately here.

The practical problem is that doing this properly will require a much better set of scripts than I currently have: what I’m presenting here is only a small corner of the dataset (forward contacts for ch and sh), executed very imperfectly (partly by hand). But hopefully it’s a step in the right direction and others will take it as an encouragement to go much further.

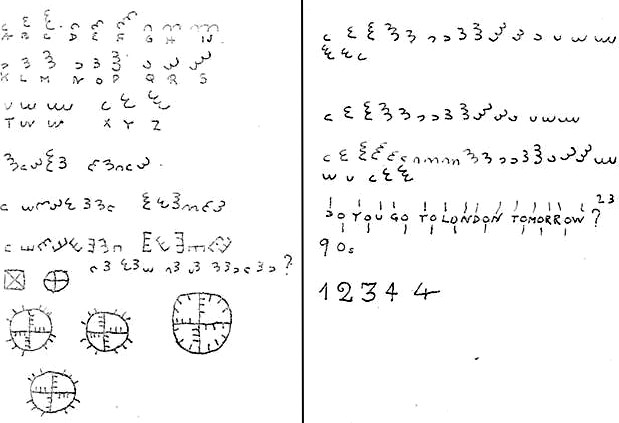
Note that I used Takahashi’s transcription, and got a number of unmatched results which I counted as ??? values. These may well be errors in the transcription or errors in my conversion of the transcription to JavaScript (which I did a decade ago). Or indeed just bit-rot in my server, I don’t know.

A ch vs B ch, Forward Contacts

A ch
(cho 1713)
—– of which (chol 531, chor 400, chod 196, chok 130, cho. 113, chot 94, chos 50, chom 28, choi 20, choy 18, chop 14, chof 12, choe 11, choa 7, choc 6, choo 5, cho- 4, chon 4, chog 2, cho??? 69)
(che 918)
—– of which (cheo 380, chey 229, chee 156, chea 64, chek 30, chet 19, ched 12, ches 8, chep 7, cher 2, cheg 1, chef 1, che. 1, che* 1, che??? 7)
(chy 544)
(cha 255)
(ch. 112)
(chk 60)
(chd 35)
(cht 31)
(chs 21)
(chch 5) (chp 5) (chsh 4) (chm 2) (chi 2) (chc 2) (chf 1) (chl 0) (chn 0) (chr 0) (chs 0) (ch- 0) (ch= 0)

B ch
(che 3640)
—– of which (ched 1482, chey 597, cheo 565, chee 537, chek 119, chea 82, ches 55, chet 42, chep 25, chef 15, cher 4, cheg 4, che. 2, chel 1, che??? 117)
(chd 725)
(cho 633)
—– of which (chol 200, chod 123, chor 83, chok 65, chot 44, cho. 34, chop 7, chos 22, choa 10, chop 7, choy 4, choe 4, chof 4, choo 4, choi 2, cho= 1, cho??? 26)
(ch. 403)
(chy 331)
(cha 185)
(chk 84)
(chs 50)
(cht 38)
(chp 20)
(chch 6) (chc 6) (chsh 5) (chf 2) (chi 0) (chm 0) (chl 0) (chn 0) (chr 0) (chs 0) (ch- 0) (ch= 0)

Observations of interest here:

  • A:cho = 1713, while B:cho = 633
  • A:chol = 531, while B:chol = 200
  • A:chor = 397, while B:chor = 83
  • A:che = 918, while B:che = 3640
  • A:ched = 12, while B:ched = 1482
  • A:chedy = 7, while B:chedy = 1193
  • A:chd = 35, while B:chd = 725
  • A:chdy = 21, while B:chdy = 504

As an aside:

  • dy appears 765 times in A, 5574 times in B

A sh vs B sh, Forward Contacts

A sh

(sho 625)
—– of which (shol 174, sho. 143, shor 105, shod 77, shok 32, shot 22, shos 11, shoi 9, shoa 6, shoy 5, shoe 4, shom 4, shop 4, sho- 1, shof 1, shoo 1, sho??? 26)
(she 407)
—– of which (sheo 174, shee 84, shey 81, shea 20, she. 19, shek 12, shes 8, shed 3, shet 2, shep 1, sheq 1, sher 1, she??? 1)
(shy 153)
(sha 58)
(sh. 39)
(shk 13)
(shd 7)
(shch 6)
(sht 5)
(shs 3)
(shsh 1)
(shf 1) (everything else 0)

B sh

(she 1997)
—– of which (shed 734, shee 386, shey 334, sheo 286, shek 78, shea 37, shet 18, shes 15, she. 13, shep 6, shef 5, shec 2, sheg 2, she* 1, shel 1, sher 1, she??? 79)
(sho 284)
—– of which (shol 89, shod 59, shor 43, shok 24, sho. 23, shot 8, shos 8, shoa 5, shoi 5, shoe 3, shof 2, shoo 2, shoy 1, shop 1, sho??? 11)
(shd 161)
(sh. 136)
(shy 104)
(sha 67)
(shk 35)
(sht 13)
(shs 12)
(shch 6)
(shsh 1)
(shf 1) (everything else 0)

Observations of interest here:

  • A:sho = 625, while B:sho = 284
  • A:shol = 174, while B:shol = 89
  • A:shor = 105, while B:shor = 43
  • A:she = 406, while B:she = 1997
  • A:shed = 3, while B:shed = 734
  • A:shedy = 2, while B:shedy = 629
  • A:shd = 7, while B:shd = 161
  • A:shdy = 3, while B:shdy = 100

Final Thoughts

The above is no more than a brief snapshot of a corner of a much larger dataset. Even here, a good number of the features of this corner have been discussed and debated for decades (some most notably by Prescott Currier).

But given that there is no shortage of EVA ch, sh, e, d in both A and B, why are EVA ched, chd, shed, and shd so sparse in A and so numerous in B?

It’s true that dy appears 7.3x more in B than in A: but even so, the ratios for ched, chedy, shed, shedy, chd, chdy, shd and shdy are even higher (123x, 170x, 244x, 314x, 20x, 24x, 23x, and 33x respectively).

Something to think about…

Dorabella Cipher: timeline, texts, and Keith Massey…

by ⋅ 24 Comments

There are plenty of things about Edward Elgar’s Dorabella Cipher that rarely appear in the countless gosh-wow sites that feature it on the web. And arguably one of the biggest of these is its timeline.

1886: The Liszt Fragment

The earliest instance we know of where Elgar used the ‘Dorabella’ shapes to write something down was when jotting something in the left margin of a programme for a Liszt concert at the Crystal Palace (10th April 1886). The best quality image of this fragment appears on p.134 in Craig Bauer’s magisterial “Unsolved!”, which I reproduce here:

This contains a fair few repeated shapes, which would be good grist for the cryptanalytic mill were the fragment not so darned short:

Though Anthony Thorley claimed to have ‘decrypted’ this fragment in (or before) 1977 as “GETS YOU TO JOY, AND HYSTERIOUS”, this looks just plain wrong to Bauer (and to me). This is not only because none of the repeated letter usages line up, but also because it’s basically the wrong length (Thorley’s phrase is 25 letters long, while the fragment is made up of eighteen shapes plus a terminal dash).

If this Liszt fragment is a cipher, I’m sure Big Data people wouldn’t have to try hugely hard to build up a list of all 18-letter English letter sequences with the same aBCDeCfgBhiDCBijkl pattern. Perhaps looking for matches for the 13-letter stretch from BCD to DCB might be a productive exercise?

At the same time, it is tempting to wonder whether Elgar was using these shapes as some kind of idiosyncratic musical notation. However, even though the eighteen-glyph-plus-hyphen fragment appears in the margin beside an eighteen-note arpeggiated melody (Liszt’s “allegretto pastorale” motif, which appears as “an independent episode” according to the programme notes), it has none of its musical symmetry.

So: even though the Liszt fragments looks as though it really ought to be a simple cipher (and, moreover, a simple cipher that Elgar had without any real doubt used many times before), none of the claimed decryptions put forward for it make much sense. It’s possible Elgar was using his own brand of self-pleasing nonsense verbiage but… this is as far as we can get.

1897: The Dorabella Cipher

According to her 1937 book “Edward Elgar: Memories of a Variation”, a young Dora Penny’s first met Edward Elgar on 6th December 1895. Elgar’s wife was an old friend of Dora’s stepmother, and so the couple had come to visit. Elgar and Dora talked, but not about music: rather, he wanted to know about Wolverhampton Wanderers (the club was close to the Penny’s house).

All the same, he did sit down at the piano in the drawing room before luncheon, where Dora turned over the pages for him. This proved to be a challenge, as “[w]hen it came to playing from his own manuscripts you often saw nothing but a few pencilled notes and a mark or two, when he was playing something tremendous – full orchestra and chorus perhaps“, though over time she did become “rather clever at it“.

Elgar first got to see a football match with Dora on 17th October 1896. He subsequently “was much taken with the names of some of the players – particularly Malpas. […] I have known him say when we met: ‘There you are. How’s Malpas?’ – a question I was not always able to answer.“

Her book reproduces a letter she received from Elgar with a distinctive red ‘E’ seal: all of which I think gives as close a representation of the likely content of the Dorabella Cipher as can reasonably be hoped for:

Forli Malvern March 4 [1897]
Dear Miss Penny
Here is some locomotive learning; so much nicer than mouldy music.
Alice tells me you are warbling wigorously in Worcestor wunce a week (alliteration archaically Norse).
I am very glad, but on second thoughts, as I have never heard you sing I am not sure: but perhaps some day if you are not rushing away I might arrange to show you over the Cathedral organ, K. John’s tomb and the Dane’s skin: (the Dane is dead).
By the way I have taken to ‘die-sinking’ as a recreation: here on the back of this is my parcel-post seal: I have to register all my MSS & they will not give a receipt unless they are sealed: so I put this on that my works may be Esily distinguished.
Kindest regards to everybody
Believe me sincerely yours
EDWARD ELGAR

The Dorabella Cipher is dated July 14 [18]97 and, if you haven’t already seen it a thousand times or more, looks like this:

This was “the third letter [Dora Penny] had from him, if indeed it is one“: so the March 4 letter was only one of two Elgar had previously written to Dora.

As to what the Dorabella Cipher says: I’ve previously (in 2013) speculated whether the first two words might be, just as with the March 4 letter, FORLI MALVERN. And the obvious suggestion that Elgar might have also included the phrase “How’s Malpas?” is entirely possible, though untested.

All the same, I’d point out that the general character of the glyph shapes seems to change on the third line. That is, the shapes lose their variety, and become visually monotonous, bland, repetitive, even dull. It’s as though Elgar kind of lost momentum, and stopped wanting to sustain the joke. Much as I have suggested with the famous unsolved Zodiac Z340 cipher (where the top half and bottom half have different statistical profiles / patterns), I do wonder whether we might be seeing two different things grafted together here, i.e. that the third line is quite different in nature from the first two. Just a thought.

Note: it was September 1898 when Edward Elgar first called Dora Penny “Dorabella” (as a quotation from Mozart’s Così fan tutte): so the one word we should not expect to see in the Dorabella Cipher is ‘Dorabella’.

1924 or later: the Marco Elgar Cipher

Yet another place where the rotating e/ee/eee letter-shapes appear in Elgar’s papers is where he uses it as a simple pigpen-style cipher:

This we can date as having been written not before 1924, because the plaintext refers to “MARCO ELGAR”, the name of Elgar’s beloved spaniel, and who was born on 27th May 1924 (a picture of his grave is here).

While the most obvious interesting thing about this it doesn’t work for the Dorabella Cipher, there is something about this sheet that gives me the impression that what Elgar is trying to do is to reconstruct his cipher system. It is hardly a coincidence, I would say (apologies to Thomas Ernst) that another phrase enciphered on this same page is “A VERY OLD CYPHER”.

Given the roughly thirty years’ difference between the Dorabella Cipher and the Marco Elgar cipher (and the absence of any other similar letter-shapes in Elgar’s generally quite well-preserved writings), perhaps it was something he amused himself with as a young man, but which he had by the age of about 70 (he was born in 1857) just plain forgotten.

Perhaps the circular shape on this page is some kind of E-based mnemonic (i.e. that the letters of the alphabet were arranged around), but which had slipped his mind. Certainly, you can see the letter E concealed in it without much difficulty, so perhaps that was part of the game?

Undated: The Cryptogram Card

Our final Elgarian cipher shapes first appeared in Craig Bauer’s “Unsolved!”. These are on a card marked “Cryptogram” (hence “The Cryptogram Card”), but are undated:

Though the writing is tiny, there are two main runs of eee-shapes: in the one just above the word “Cryptogram”, the triple curve shape rotates around, as if (as Craig points out) it is doing a gymnastic forward roll. In the run just below the word “Cryptogram, the three sizes of right facing ‘e’ appear in descending order, followed by the next rotation round. There are also a couple of cipher letters at the top.

What we see here are more like pen trials than cryptograms: so in almost all senses there’s really nothing of importance here.

Recent Dorabella Theories

Plenty of clever people – not just Eric Sams and Tony Gaffney – have already put forward their thoughts about (and their attempted decryptions of) the Dorabella Cipher. Needless to say, not more than one of them can be right at the same time. 🙂

But the list of attempts to explain it keeps getting longer. When Klaus Schmeh blogged about the Dorabella in 2018, one of the commenters (Thomas Ernst) put forward – at some length – his notion that Dora Penny might herself have faked all Elgar’s ciphers. This is an interesting suggestion: Dora certainly had full access to Elgar’s archives for decades, so clearly had opportunity – and I can see what he’s getting at when he draws a parallel between Dora Penny’s book and Bettina von Arnim’s (1835) “Goethes Briefwechsel mit einem Kinde”, which contains numerous stories about Goethe falsely adjusted to bring Bettina herself into the foreground (think of it as a kind of literary Forrest Gump).

But I think Ernst is being far too literal when he draws negative conclusions from the way that the post-1924 Marco Elgar page “A VERY OLD CYPHER” alphabet does not work for the Dorabella Cipher. His reasoning (unless I’ve misunderstood it) is that because the two are inconsistent, at least one is not genuine. And he then goes on to argue that if one is not genuine, there’s no reason to think that they are both not genuine.

I would agree that the two are indeed inconsistent. However, the rather different inferences I draw from the Marco Elgar page are that (a) in it, Elgar gives the impression that he was trying to reconstruct a cipher system he had used as a much younger man; and (b) there was some kind of underlying symmetry to the letter-to-glyph assignments in that cipher that he simply could not remember.

So, although there are good reasons we should all be aware of the inconsistency between accounts, Ernst’s move to a full-fat hoaxed-by-Dora theory seems somewhat pessimistic and extravagant to me.

As an aside, I think it would be a good exercise to analyze the Liszt Fragment and the Dorabella Cipher to see if they are consistent or inconsistent with each other (e.g. by comparing letter contact tables etc).

Another commenter (ShadowWolf) used the same Klaus post to put forward his/her own Dorabella decrypt (which, perhaps almost inevitably, involves a cipher-style first pass and a this-is-what-Elgar-really-meant-by-that interpretational second pass, Eric Sams-style):

Plaintext:
PBS AFT DALYRENCE MEET B BECO YOUR IDEDTD ALWASE
E STUNDER E THINC OLL OR IS IT HIS CH GUISE
THNIC ABU IT ACOA

Message:
Problems after dalliance meet is because your identity always
a stutter I think all or is it his charming guise?
Think about it acolyte.

In a similar vein, Cipher Mysteries readers may possibly recall that I posted about Allan Gillespie’s Dorabella Cipher theory back in 2013: his (somewhat hybridized) theory was that the plaintext began “ForlE Malvern Link”, the encipherment used a Vigenère cipher system, but (not entirely unlike Thomas Ernst’s theory) it had been “concocted by someone other than Elgar (possibly in the run-up to WWII when GC&CS were recruiting; possibly with Dora Powell’s connivance, more likely not)“.

Another Dorabella solver is Mark Pitt, a Cleveland police officer “with an MA in crime patterns” who has already had the oxygen of publicity in the Times, Daily Mail, Daily Telegraph and Guardian (to name but four). Pitt has also claimed to have decrypted the Liszt fragment: his solution for both seems to be based around Schooling’s cipher that Elgar famously cracked, with the key “PRUDENTIA”. I suspect Pitt has a (not very active) Twitter account, but that’s just my hunch. A (paywalled) Telegraph article on him from early 2019 is here.

The Two Massey Observations

Finally, a very different take on the Dorabella Cipher has been put forward by Keith Massey in an 11-minute YouTube video from 2017 (but which I only stumbled upon recently), based on two very specific observations.

His first observation is that the Dorabella Cipher contains long sequences of glyphs where no two adjacent glyphs have the same number of loops. Specifically, the first line has a sequence with 12 loop-number-differing glyphs in a row; the second line has a sequence with 9 loop-number-differing glyphs in a row; while the third line has a sequence with 8 loop-number-differing glyphs in a row; all three sequences are near the start of their respective lines. Massey’s control experiments (two of them, which one might reasonably argue is a little bit lightweight) each yielded a single maximum of only 5 or 6 loop-number-differing glyphs in a row. (A more Oranchek-esque researcher would surely have done the experiment by anagramming the Dorabella a billion times over, but I suspect the results would have been not wildly dissimilar.)

Massey’s second observation is that the Dorabella Cipher contains way too many pairs of opposed symbols (i.e. where a glyph is immediately followed by a glyph with its same basic shape but where that second shape is rotated by 180 degrees). Massey calculates that this should on average ~5.3 times for 87 characters, but it instead occurs 12 times.

If we assume all 24 shapes are equally likely to occur (which isn’t true) across the Dorabella’s 87 characters, the probability of 13 exact opposites occurring is ((1/24)^13)*((23/24)^(87-1-13)), which Google tells me is 5.1046414e-20 (i.e. about one in twenty billion billion). Again, a more realistic (i.e. Oranchakian) way of doing this would be to anagram the ciphertext billions of times and see how often twelve exact opposites occur (i.e. using the actual distribution rather than an ideal [perfectly flat] distribution). My prediction here is that the probability there would still be no higher than one in a billion billion, so I believe this too is likely to be a statistically significant result.

Massey thinks the final nail in the Dorabella’s cryptological coffin is that these two patterns don’t overlap: he believes that

Massey’s overall conclusion is that that Elgar created the Dorabella Cipher as nonsense text to resemble a ciphertext as a joke on Dora Penny, but that this nonsense text eventually escaped to become a joke on all of us.

Even if you disagree with the full strength of his conclusion, I suspect these two Massey observations will prove difficult for anybody proposing a simple MASC as a solution (albeit typically with an interpretational second phase) to satisfactorily account for what we see in the Dorabella Cipher.

Thoughts and Conclusions

I have to say that I’m very largely with Keith Massey here, insofar as he is pointing out statistical features of the Dorabella Cipher that are highly improbable. It is almost impossible not to see that these sit awkwardly with the traditionalist (one might call it ‘Samsian‘) reading of the cryptogram’s system as a pigpen-style simple substitution cipher applied to an idiosyncratic Elgarian nonsense-wonsense text. It would be good if Massey’s observations were to be confirmed in a more statistically robust manner, but I would be surprised if the actual results proved to be vastly different.

My own suspicion (just as in 2013) remains that the Dorabella Cipher may turn out to be a stegotext visually concealing a guessable personal message (e.g. “FORLI MALVERN”) rather than a cryptotext mathematically concealing a plaintext. And I believe this is far from inconsistent with Massey’s observations, though only for the left hand half of the three lines.

But even so, I’m really not at all convinced that his observations hold true for the Liszt Fragment, which I believe was written in the same “VERY OLD CYPHER” that Elgar was trying to reconstruct in the Marco Elgar page.

So there is perhaps still work to be done on a genuine Elgar cipher here, even if Massey has indeed managed to nail down the Dorabella MASC coffin (and all credit to him if he has!).

Chloe Delancy (Thomas Beale Jr’s Mother)…

by ⋅ 2 Comments

Given that I’ve paid my findmypast subscription (and that money’s not coming back any time soon), I thought it might be interesting to look at the records it holds for Thomas Beale Jr‘s mother Chloe Delancy / Delancey. We know a fair bit about Thomas Beale Sr, so why not find out more about his mother?

Chloe Delancy / Delancey

As far as I can see, “Cloe Delancy” only appears in Botetourt County in the 1810 and 1820 US census records. This would seem to imply that she married, moved, or died before the 1830 census – given that there are plenty of holes in the census records, it’s sensible to be at least a bit defensive.

In the 1810 census records, she is apparently living alone (“Number of free white females age 26-44” = 1) – every other column is blank. (Hence it would seem that Thomas Beale Jr may not have been living with her then.) Other than being in Botetourt County VA, no location is given.

In the 1820 census records, there is one “free white female age 45 and up” (presumably her), one “free white female age 10-16”, and one “free white female under age 10”. The location is noted in the margin as “Fin.”, which is without any doubt Fincastle.

There’s no obvious sign of her in the 1830 Census, yet that was the year that the case Delancey vs Beale was in the Supreme Court in Louisiana, so she was presumably still alive then (unless you know better?).

(Note that there is an online genealogy mentioning a Chloe Emaline Delancy b. 1834 Rockingham NC to William D. Delancey (1785-1860) and Catherine [nee Roach] (1799-1860): but this person seems entirely unconnected.)

Virginia Cloes / Chloes?

The 1830 Census has a Chloe Switcher living in Botentourt County, but she is a F 30-40 living with a M 15-20. Similarly, the 1840 Census has a Cloe Switzer, but she is a F 40-50 living with a M 20-30: I think it’s a pretty safe bet that the two entries refer to the same person, and that this probably isn’t Chloe Delancy.

Broadening the search a little, there are eight women called Cloe in Virginia listed in the 1830 Census: Cooper, Masters, Myho, Powelson, Simmons, Whichard, and Withers (though note that these are all the head of their household).

  • Cloe Cooper: 1 x F under 5, 1 x F 20-30, 1 x F 50-60
  • Cloe Masters: 1 x M under 5, 1 x M 5-10, 2 x M 15-20, 1 x M 20-30, 1 x F 2-30, 3 x F 30-40, 1 x F 60-70
  • Cloe Myho (actually Mayho): no details of household supplied
  • Cloe Powelson: 1 x M 10-15, 1 x M 15-20, 1 x M 20-30, 1 x F 10-15, 1 x F 15-20, 1 x F 40-50 [also in 1840 and 1850 censuses]
  • Cloe Simmons: 1 x F 5-10, 1 x F 50-60
  • Cloe Whichard: 1 x M 30-40, 1 x F 20-30, 1x F 50-60
  • Cloe Withers: 1 x M 30-40, 1 x F 15-20, 1 x F 70-80

Similarly, there are sixteen women called Chloe in Virginia listed in the 1830 Census: Atkins, Buske, Cheshire, Coleman, Ellison, Gaskins, Goodrich, James, Lunsford, Mifflin, Mills, Pitman, Powell, Sitcher, Thomas, and Vanlandingham.

  • Chloe Atkins: 1 x M 20-30, 1 x F 15-20, 2 x F 50-60 [also in the 1840 census]
  • Chloe Buske: 1 x M 20-30, 3 x F 20-30, 1 x F 50-60
  • Chloe Cheshire: 1 x M under 5, 1 x M 15-20, 1 x F under 5, 1 x F 10-15, 1 x F 20-30, 1 x F 50-60 [also in the 1810 census]
  • Chloe Coleman: 1 x M under 5, 1 x M 20-30, 2 x F 5-10, 2 x F 30-40, 1 x F 60-70
  • Chloe Ellison: 1 x F 50-60 [also in the 1810, 1820 and 1840 censuses]
  • Chloe Gaskins: 1 x M under 5, 1 x M 5-10, 1 x M 10-15, 1 x F 30-40 [also in the 1850 census]
  • Chloe Goodrich: 1 x F 60-70
  • Chloe James: 1 x F 20-30, 1 x F 70-80
  • Chloe Lunsford: 2 x F 15-20, 1 x F 50-60
  • Chloe Mifflin: no details given
  • Chloe Mills: 1 x M 10-15, 1 x M 15-20, 1 x M 20-30, 2 x F 5-10, 1 x F 15-20, 1 x F 20-30
  • Chloe Pitman: 1 x F 50-60 [also in the 1840 census]
  • Chloe Powell: 1 x M 5-10, 1 x F 5-10, 1 x F 30-40
  • Chloe Sitcher/Switcher/Switzer: 1 x M 15-20, 1 x F 30-40
  • Chloe Thomas: 1 x F 20-30, 1 x F 60-70 [also in the 1820 census]
  • Chloe Vanlandingham: 1 x M 10-15, 1 x F under 5, 1 x F 10-15, 1 x F 15-20, 1 x F 40-50

Note that Cloe Cooper is also in the 1840 Census, but listed as F 80-90.

Any Other Mentions?

There is one possible mention I found, which is in the Annals of Southwest Virginia 1769-1800 (Lewis Preston Summers, 1929), p.465. There, the entry for 10th February 1796 in the minutes of the County Court mentions that the grand jury presented “Isaac Dawson and Chloe Delaney for living in an unlawful way”.

Thoughts on the US Census

I have to say I was expecting to find a little more than I did. It may be that we now have a weak indication that Chloe Delancey had two younger daughters we were (or, at the very least, I was) previously unaware of: but the limitations of the census data mean that we have (I think) no obvious paths to go down to find their names.

Has anyone got any better information on Chloe Delancey and/or her possible two daughters than this?

More about Triantafillos Balutis…

by ⋅ 2 Comments

I thought my last post had gone through pretty much all the sources available online relating to Triantafillos Balutis, the Melbourne waiter who the PRO Victoria flagged as possibly being the mysterious “Balutz” at Christos Paizes’ Lonsdale Street baccarat club. But, thanks to the almost endless spelling variations of his names, it turns out I was wrong.

Which is good!

1930 Naturalisation Certificate

For a start, the NAA has a file marked “Treantafellous BALUTES – Naturalisation certificate” (NAA A1, 1930/1546), which is the correspondence and certificate (“A.A. 6302”) relating to Triantafillos Balutis’ naturalisation application.

From this, we learn that:

  • his address was Victoria Hotel, 404 Bourke Street, Melbourne;
  • he had no wife or children;
  • he had placed advertisements for his naturalisation application in the Argus and Age, both of the 24 Jan 1930;
  • he was 5ft 5in, black hair, brown eyes, small mole on right cheek;
  • he was born on 5 Aug 1886, in Cavalla in Greece;
  • his father was Dameanos Balutes, and his parents were both Greek;
  • he arrived in Melbourne from Greece on the 16 Feb 1923 on the S.S. Ormonde;
  • after leaving Greece but before coming to Australia, he lived in the USA for eight years;
  • he was a café proprietor, who had been running a café at 426 Bourke Street, Melbourne for four years and five months; and
  • he was represented by Messrs. Luke Murphy & Co, Solicitors, 422 Bourke Street, Melbourne.

The general remarks section on the form asserts:

Applicant has been established in business in Bourke St. at the Canberra Café during the past 4½ years. He has opened up a further business at Warrnambool for the manufacture of cheese, which he proposes to export to Egypt & U.S.A. Applicant is of the keen type of business man & gained a good business knowledge during his residence in the U.S.A. for about 8 years. There is nothing known against applicant.

His three referees were two householders and a police officer:

  1. Donald Mackintosh, Gun Maker, of 2 Thistle Street, Essendon
  2. Horace Govett James, Business Manager, of 3 Sunnyside Grove, Bentleigh
  3. Sidney James Kirby, Constable of Police, of Russell Street, Melbourne

From this, we learn that – despite the apparently contradictory evidence presented in the previous post – all the evidential threads tie together, i.e. there was only one Triantafillos Balutis, even though his date of birth seems somewhat uncertain. His full name would therefore have been Triantafillos Dameanou Balutis.

Note that when he was born in Kavala, it was part of the Ottoman Empire (Greece absorbed it in 1912 during the Balkan War). So his nationality at the time of his birth was Turkish, but later became Greek: hence he was both Greek and Turkish, depending on how you asked the question. Nationality can be quite a fluid thing!

George Vrachnas & Jack Lenos

The NAA lists two other documents relating to him. The first, dated 1930, is item NAA: A10075, 1930/21 (item barcode: 3140391) is “BALUTES Treantafellous versus VRACKNAS George; LENOS Jack”, and relates to a cause (complaint) brought by one party against another before a single judge. (Not yet online.)

According to findmypast, George Vrachnas was born in 1890: and had a restaurant in the ground floor of Traynor House, 287 Elizabeth street. Though Vrachnas & Lenos appear in a number of other cases that appear in Trove (e.g. Wolff vs. Vrachnas and Lenos; Boyd vs. Vrachnas and Lenos; Palmer vs Vrachnas and Lenos, etc, while 1932 saw the inevitable Vrachnas vs Lenos), I so far haven’t found anything relating to Balutes vs Vrachnas and Lenos.

We can see a separate case being taken against the pair in 6 Nov 1931:

IN THE COURT OF PETTY SESSIONS, HOLDEN AT WATER POLICE OFFICE, SYDNEY. No. of Writ. 5993 of 1931. No. of Plaint, 5680 of 1931. THE NATIONAL CASH REGISTER CO. OF A/SIA, LTD., Plaintiff; and GEORGE VRACHNAS and JACK LENOS, trading as Vrachnos and Lenos, 215 Oxford-street, Sydney, Defendant. UNLESS the amount of £14/17/11, together with all fees due herein be paid at or before the hour of noon To-day, Friday, the sixth day of November, 1931, the Bailiff will sell by Public Auction, at Water Police Office, the Right, Title, and Interest of the defendants in goods which are the subject of Conditional Bill of Sale dated 16th July, 1930, No. 13779. last renewed 9th September, 1931, between George Vrachnas and John Lenos (Mortgagors) and John Vrachnas (Mortgagee), and the Right, Title, and Interest of the defendant George Vrachnas In goods which are the subject of conditional Bill of Sale dated 11th October, 1930, No. 19858. between George Vrachnas (Mortgagor) and A. A. Marks, Limited (Mortgagees). Dated at the Court of Petty Sessions abovementioned, this twelfth day of October. 1931.

Incidentally, Trove mentions that Gwendoline Vrachnas was charged in June 1932 with being a manager of a common gaming house in Elizabeth-street, Sydney, in relation to “the sale of share tickets in the State Lottery”.

As a final aside, there’s an oral history recording of George Vrachnas online here, reminiscing about his life. In one part he mentions the effect of the Depression upon his business (suddenly none of the businesses renting from him could pay their rents, and the whole setup collapsed), which was the point in his life when his fortunes dramatically changed.

Police Records

The last of the NAA records is simply titled “Treantafellous Balutes” (NAA: B741, V/7104, Item barcode: 1140692, Location: Melbourne), and contains (or, at least, seems to contain) details of his Victoria police record from 1930 to 1949. Even if Balutis wasn’t in the Victoria Police Gazette for 1944 / 1945 / 1946, it would seem that there was still police interest in his activities.

The B741 series:

[…] comprises files relating to the investigation of all criminal offences committed against the Commonwealth, the contravention of Commonwealth Acts or of State Acts committed on Commonwealth property; the pursuit of recalcitrant debtors to the Commonwealth; and inquiry into the whereabouts of persons requested to be traced by government departments, organisations such as the Red Cross, International Tracing Service, Australia House, private persons or by diplomatic or consular representation. Investigations carried out at the request of government departments include areas such as narcotics trafficking, impersonation, bribery, “forge and utter”, ships’ deserters, enemy aliens in wartime, prohibited immigrants, naturalisation, and rape on Commonwealth property. In most instances a separate file was raised for each particular case requested to be investigated.

It therefore may well also be that Balutis appears in Victoria’s B745 series (because, as it says, “No items from the series are on RecordSearch“):

Name (offenders) index cards to: (1) Correspondence files, single number series with “V” (Victoria) prefix, 1924 – 1962 (2) Correspondence files re Police investigations, annual single number series, 1963 –

The series is the name index to all persons committing an offence against the Commonwealth and/or contravening Commonwealth legislation or State legislation on Commonwealth property, persons whose whereabouts have or are being investigated, and up until 1963, recalcitrant debtors to the Commonwealth.

The Shadow of the Depression

The Depression cast a deep, malign shadow over the life of George Vrachnas, and it seems to have had the same effect on Triantafillos Balutis.

Even though he applied for his naturalisation in January 1930, that was right at the end of the good times. Before that, you can see from Trove that Vrachnas’ café had held regular social meetings and dances, often raising money for war veterans: but now the 1920s were gone, and a different kind of economic reality was in place.

For Balutis, I think you can see the same thing via the advertisements in Trove, from the 2 Jan 1930 (just before his naturalisation)…

Waitress, experienced, start at once, no Sunday work. Canberra Cafe, 426 Bourke st.

…to the 10 Feb 1930 (just after his naturalisation)…

WAITRESS, 16 to 18 years, ready to start, permanent. Canberra Cafe, cr. Lonsdale and Swanston sts.

…to, alas, 13 Dec 1930…

THURSDAY, 18th DECEMBER. At Half-past 2 o’Clock. On the Premises, 426 Bourke-street, MELBOURNE. Under Power of Bill of Sale No. 173,535, instructed by Mr. A. H, HILL, 11 Elizabeth-Street, Melbourne. COMPLETE FURNISHINGS AND PLANT OF CANBERRA CAFE. SODA FOUNTAIN, SODA WATER MACHINE, JACKSON BOILER COMPLETE With Pie Heater; NATIONAL CASH REGISTER, TOLEDO SCALES, 2 Ice Chests, Cutlery, Crockery, Glassware, &c. The Whole To Be Offered As a Going Concern. Full Particulars in Future Advertisement. R. RICHARDSON, Auctioneer, 18 Queen-street.

Whatever the relationship between Balutis and Vrachnas & Lenos was, 1930 seems to have been the year everything went wrong both in the macro-economy and in the Melbourne micro-economy. It was not only the year that Balutis became a naturalised Australian, but also the year that the Australian economy – as the phrase goes – went South.

I think it’s fair to say that a lot of dreams died that year.

What Would I Like To See Next?

As always, the archive records accessible online are only the tip of a giant evidential iceberg. So, the (non-online) documents I’d really like to see next are all held in Melbourne archives:

  1. “Treantafellous Balutes” B741 V/7104 (barcode 1140692) from NAA Melbourne (99 Shiel St, North Melbourne). All I know about this is that it covers the date range 1930-1949: beyond that, all outcomes are possible.
  2. I’d also like to know if any Balutis / Balutes / Balutz is mentioned in the B745 series. This is the set of name / offender index cards maintained by Victoria’s Investigation Branch: so if anyone had any contact with the Victoria police from 1924-1962, their card should be there. Having said that, it’s not entirely clear to me from the NAA online description whether B745 is at North Melbourne at all. Getting some clarity on this would be very good!
  3. As an aside: if it turns out that B745 is accessible, I’d also (just in case, you never know, it’s possible that, etc) really like to see the index cards of all the (T or J first initial) Kean / Keane individuals. Because if it were to turn out that any of those had been charged with nitkeeping prior to 1 Dec 1948, we might just have struck gold. 😉
  4. Finally, I’d also like to see Stelios Balutes’ death records (he died on 09 Jul 1977). According to PRO Victoria’s website, their archives hold both his will (PROV ref: VPRS 7591/ P4 unit 757, item 836/255) and his probate records (PROV ref: VPRS 28/ P8 unit 494, item 836/255), both of which I’d like to see. I’d guess that they are stored together (because they share the same item number), but you never can tell with archives. These are held at PRO Victoria’s North Melbourne site (also at 99 Shiel St, North Melbourne).