Might Paul Olum have been the person behind Richard Feynman’s Ciphers?

A fascinating email from long-time Cipher Mysteries commenter Paul Relkin has alerted me to a pair of ciphers by mathematician Paul Olum (1918-2001), who knew Feynman at Princeton, and then worked with him at Los Alamos. Sure, you can read Olum’s Wikipedia page, but perhaps the best person to describe him is Richard Feynman himself (in “Surely You’re Joking, Mr Feynman”):

They were all giving me problems and I was feeling great, when Paul Olum walked by in the hall. Paul had worked with me for a while at Princeton before coming out to Los Alamos, and he was always cleverer than I was. For instance, one day I was absent­mindedly playing with one of those measuring tapes that snap back into your hand when you push a button. The tape would always slap over and hit my hand, and it hurt a little bit. “Geez!” I exclaimed. “What a dope I am. I keep playing with this thing, and it hurts me every time.”

He said, “You don’t hold it right,” and took the damn thing, pulled out the tape, pushed the button, and it came right back. No hurt.

“Wow! How do you do that?” I exclaimed.

“Figure it out!”

For the next two weeks I’m walking all around Princeton, snapping this tape back until my hand is absolutely raw. Finally I can’t take it any longer. “Paul! I give up! How the hell do you hold it so it doesn’t hurt?”

“Who says it doesn’t hurt? It hurts me too!”

I felt so stupid. He had gotten me to go around and hurt my hand for two weeks!

So Paul is walking past the lunch place and these guys are all excited. “Hey, Paul!” they call out. “Feynman’s terrific! We give him a problem that can be stated in ten seconds, and in a minute he gets the answer to 10 percent. Why don’t you give him one?”

Without hardly stopping, he says, “The tangent of 10 to the 100th.” I was sunk: you have to divide by pi to 100 decimal places! It was hopeless.

One time I boasted, “I can do by other methods any integral anybody else needs contour integration to do.”

So Paul puts up this tremendous damn integral he had obtained by starting out with a complex function that he knew the answer to, taking out the real part of it and leaving only the complex part. He had unwrapped it so it was only possible by contour integration! He was always deflating me like that. He was a very smart fellow.

Olum I

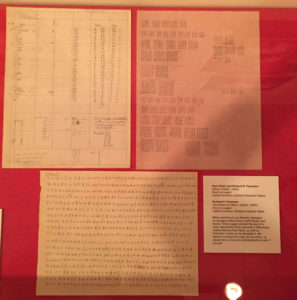
But back to the recently unearthed pair of Paul Olum’s ciphertexts. The first one surfaced in 2018 at a Caltech exhibition to celebrate what would have been Feynman’s 100th birthday, and then bubbled through into a Reddit post in 2019. This (marked as “Olum I”) included Feynman’s notes on it (character frequency counts etc):

Feynman’s conclusion was that it was probably a “simple substitution [cipher] with some rearranging. The rearranging is not very complete“, and suggested some possible substitutions: “N -> T, G -> K, X -> N, F -> C, D ->G, (U -> W)?“. Though, as seems to have been the case fairly often, he didn’t quite get the better of Olum here.

If you want to have a tilt at Olum’s first windmill, the Reddit poster (“V sbyybjrq gur ehyrf”) transcribed its 744 characters as follows:

VEWLJ NBBEL QFWSX HBUSW AIBYQ AEQSI GHOVN BSNBV LNWXA BIQIU BGBIC YQFXC EVBWX WBSNG WVEVL HWDHB ILMHB LNSGB HSNXS XBHLQ CBOCS OBVWM XFNCW PAGGN EUWGA IBVWI FYWFG GQFEW MPQIX XWSEW VIHAB EBWJX OHAFQ LBBNI BHAIV JNSHC WXPCY UGGOB DWAXB HBWIN XWSNJ GWVAF OXBLM WAEBP BBWXC RBWBV LHIJA JINOW XDBIB QCGYW FXHCQ AIBCW NGCSC SHBNA VIEWD HIBLH EBVVY YSLRQ PQVCQ IWXQE DQBIW XWEAP BHVWS BSBWX VAVHB WFPUH BYWVN BYIOQ WAIFY QDXDB ICLBW YCNEA IBWIN BBWAA CIQIC VWIXQ VCBLH XIBVL AHMFO BXSIX OQBUE PCOVA WMOFV NCWAP GGNEE UWAIW XAWAE EWOLE WESHW FXHEG HCIVB HSWJO ILAWF NDDFQ WDHIL VHBBW AIQBI OUXWS BNIGW VXVQD BVAWI FGWXN VWEPU HYWDB HIMLH BLPNM WVHYP BYWBH AMFXX OSCVN BHCWV NYBIO QWAVI YBQXD LBNDV WCCGN AABXQ VWDBH EILHJ BLNVW VBHAF XOBCB MYWIN SBVOQ WLOHC GGWFB BNSYM DQUBX WSNGB WVWAI VGXHB OJWDB HIBVL HMWBI HIWJG NBFBH DQBIW IBOBJ OHUHV YLQMY WSNSI DFWDD VWEWV HYDLW VWGPW SSHAB ILBWY WJLHD XXSH

Olum II

Olum II (the second ciphertext Olum passed to Feynman) looks to be a pure transposition cipher, though anyone looking at its length (227, a prime number) and hoping for a simple modulo-227 transposition is going to be somewhat disappointed (I tried this to give my suffixity metric a bit of a workout). Note that the 25th character seems to be lower-case ‘f’, though whether or not that is relevant or useful is another matter entirely. Note also that, mathematically, there’s no point doing multiple consecutive modulo transpositions (e.g. modulo 6 followed by modulo 7), because an N-mod-A transposition followed by an N-mod-B transposition is the same as an N-mod-((A x B)mod N)) transposition:

The same Reddit poster (“V sbyybjrq gur ehyrf”) transcribed Olum II’s 227 characters as follows:

EEIOL CNTPA TIILM NIHGU TIGLf OOOHR BYSCD EYGSE EIEEL MERSB ITCBA ANEIT GDSDD OURDM SIOMH ESELE DNSRR NHNIN ATONW AEDSY ROWHE DRTRA SVAWH EODES ETVIF NIEHE TOIGI ELNII TONAR THTHL EULII TAISL SUNFC EAINI ELSLT LBPSN TMTIH SDSIH TREIE NDUET HHIOM EIIAS TVHPF YGSOR NEEII ET

To be honest, I would expect Paul Olum to have rolled out some kind of funky modulo maths trickery here, so my strong suspicion is that this is likely to be a test more of mathematical cunning than of cryptological brains.

Thoughts on this, Nick?

As Paul Relkin reminded me, I once crypto-profiled the author of the Feynman challenge ciphers as being most likely “interested in snickering into his beard about having pulled the wool over Richard Feynman’s sainted eyes“. And I think you’d have to admit that Paul Olum does seem to match that description well (errrm… apart from the fact he was clean-shaven). Yet proof is wondrously hard to achieve, so for now this remains no more than an interesting possibility.

As for Olum II: nowadays, I wonder whether the right place to start on this kind of complicated challenge transposition would be by searching for it in Project Gutenberg. By which I mean:

• Get the A-Z frequency counts for the 227-letter challenge cipher
• Go through all the Project Gutenberg files, converting them to A-Z (and no spaces)
• For every 227-letter stretch in each file, compare the A-Z frequency counts against the cipher’s frequency counts
• Display all the exact (and very close) matches you find (though right now I have no feel for how many that would be)

That is, I’m wondering whether you might be able to use Project Gutenberg to brute force an answer to a challenge transposition cipher without actually knowing how the transposition works.

This shouldn’t actually take long to calculate, and would parallelise very well. Anybody want to give this a go?